Page 48 - ISC-12

Comprehension Type

This section contains paragraphs each describing theory, experiments, data, etc.
Questions on each paragraph are given. Each question has one or more than one
correct answer among the four given options (A), (B), (C) and (D).

Paragraph for Question Nos. 1 and 2

There are five students S 1 , S 2 , S 3 , S 4 and S 5 in a music class and for them there are five seats R 1 , R 2 , R 3 ,

R 4 and R 5 arranged in a row, where initially the seat R i is allotted to the student S i , i = 1, 2, 3, 4, 5. But,
on the examination day, the five students are randomly allotted the five seats.

1. The probability that, on the examination day, the student S 1 gets the previously allotted seat R 1 , and
NONE of the remaining students gets the seat previously allotted to him/her is
3 1 7 1
(A) . (B) . (C) . (D) .
40 8 40 5

2. For i = 1, 2, 3, 4, let T i denote the event that the students S i and S i+1 do NOT sit adjacent to each
other on the day of the examination. Then, the probability of the event T 1 ∩ T 2 ∩ T 3 ∩ T 4 is

1 1 7 1
(A) . (B) . (C) . (D) .
15 10 60 5
[JEE 2018]

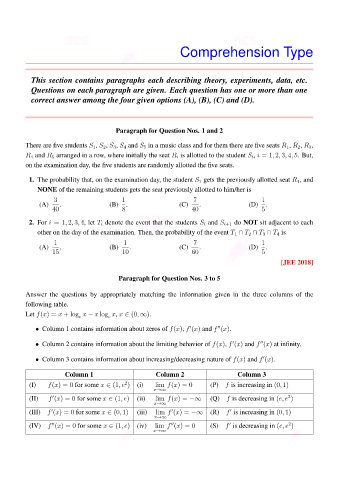
Paragraph for Question Nos. 3 to 5

Answer the questions by appropriately matching the information given in the three columns of the
following table.
Let f(x) = x + log x − x log x, x ∈ (0, ∞).
e
e
0
00
• Column 1 contains information about zeros of f(x), f (x) and f (x).
00
0
• Column 2 contains information about the limiting behavior of f(x), f (x) and f (x) at infinity.
0
• Column 3 contains information about increasing/decreasing nature of f(x) and f (x).

Column 1 Column 2 Column 3
2
(I) f(x) = 0 for some x ∈ (1, e ) (i) lim f(x) = 0 (P) f is increasing in (0, 1)
x→∞
0
2
(II) f (x) = 0 for some x ∈ (1, e) (ii) lim f(x) = −∞ (Q) f is decreasing in (e, e )
x→∞
0
0
0
(III) f (x) = 0 for some x ∈ (0, 1) (iii) lim f (x) = −∞ (R) f is increasing in (0, 1)
x→∞
00
2
0
00
(IV) f (x) = 0 for some x ∈ (1, e) (iv) lim f (x) = 0 (S) f is decreasing in (e, e )
x→∞