Page 56 - ISC-12

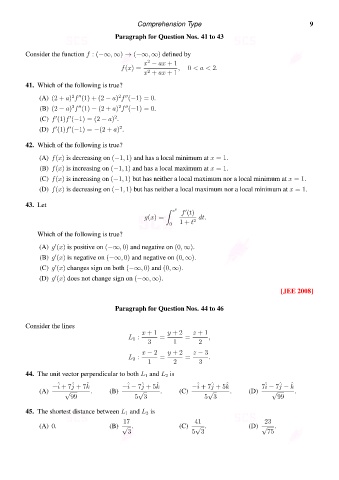
Comprehension Type Jai Baba Ki9

Paragraph for Question Nos. 41 to 43

Consider the function f : (−∞, ∞) → (−∞, ∞) defined by
2
x − ax + 1
f(x) = , 0 < a < 2.
2
x + ax + 1
41. Which of the following is true?

2 00
2 00
(A) (2 + a) f (1) + (2 − a) f (−1) = 0.
2 00
2 00
(B) (2 − a) f (1) − (2 + a) f (−1) = 0.
0
0
2
(C) f (1)f (−1) = (2 − a) .
0
0
2
(D) f (1)f (−1) = −(2 + a) .
42. Which of the following is true?
(A) f(x) is decreasing on (−1, 1) and has a local minimum at x = 1.
(B) f(x) is increasing on (−1, 1) and has a local maximum at x = 1.
(C) f(x) is increasing on (−1, 1) but has neither a local maximum nor a local minimum at x = 1.
(D) f(x) is decreasing on (−1, 1) but has neither a local maximum nor a local minimum at x = 1.

43. Let
0
e f (t)
Z x
g(x) = 2 dt.
0 1 + t
Which of the following is true?
0
(A) g (x) is positive on (−∞, 0) and negative on (0, ∞).
0
(B) g (x) is negative on (−∞, 0) and negative on (0, ∞).
0
(C) g (x) changes sign on both (−∞, 0) and (0, ∞).
0
(D) g (x) does not change sign on (−∞, ∞).
[JEE 2008]

Paragraph for Question Nos. 44 to 46

Consider the lines
x + 1 y + 2 z + 1
L 1 : = = ,
3 1 2

x − 2 y + 2 z − 3
L 2 : = = .
1 2 3
44. The unit vector perpendicular to both L 1 and L 2 is

ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
−i + 7j + 7k −i − 7j + 5k −i + 7j + 5k 7i − 7j − k
(A) √ . (B) √ . (C) √ . (D) √ .
99 5 3 5 3 99
45. The shortest distance between L 1 and L 2 is
17 41 23
(A) 0. (B) √ . (C) √ . (D) √ .
3 5 3 75