Page 69 - ISC-12

Matching-List & Matching-Matrix Type Jai Baba Ki11

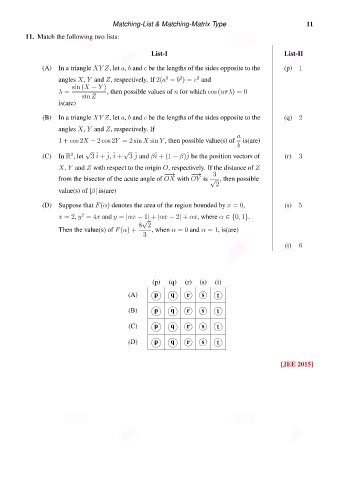
11. Match the following two lists:

List-I List-II

(A) In a triangle XY Z, let a, b and c be the lengths of the sides opposite to the (p) 1

2
2
2
angles X, Y and Z, respectively. If 2(a − b ) = c and
sin (X − Y )
λ = , then possible values of n for which cos (nπλ) = 0
sin Z
is(are)
(B) In a triangle XY Z, let a, b and c be the lengths of the sides opposite to the (q) 2

angles X, Y and Z, respectively. If
a
1 + cos 2X − 2 cos 2Y = 2 sin X sin Y , then possible value(s) of is(are)
b
√ √
ˆ
ˆ
ˆ
ˆ ˆ
ˆ
2
(C) In R , let 3 i + j, i + 3 j and βi + (1 − β)j be the position vectors of (r) 3
X, Y and Z with respect to the origin O, respectively. If the distance of Z
−−→ −−→ 3
from the bisector of the acute angle of OX with OY is √ , then possible
2
value(s) of |β| is(are)
(D) Suppose that F(α) denotes the area of the region bounded by x = 0, (s) 5
2
x = 2, y = 4x and y = |αx − 1| + |αx − 2| + αx, where α ∈ {0, 1}.
√
8 2
Then the value(s) of F(α) + , when α = 0 and α = 1, is(are)
3
(t) 6

(p) (q) (r) (s) (t)

(A) p q r s t

(B) p q r s t

(C) p q r s t

(D) p q r s t

[JEE 2015]