Page 11 - ISC-12

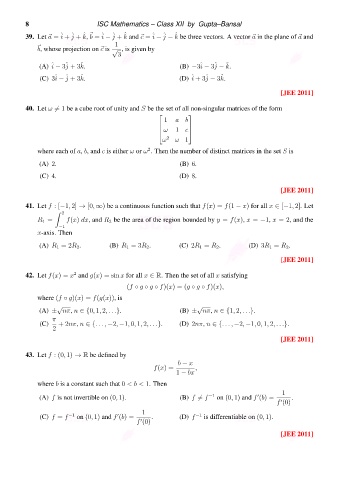
8Jai Baba Ki ISC Mathematics – Class XII by Gupta–Bansal
ˆ ~
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
39. Let ~a = i + j + k, b = i − j + k and ~c = i − j − k be three vectors. A vector ~a in the plane of ~a and
1
~ b, whose projection on ~c is √ , is given by
3
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
(A) i − 3j + 3k. (B) −3i − 3j − k.
ˆ
ˆ
ˆ
ˆ
ˆ
ˆ
(C) 3i − j + 3k. (D) i + 3j − 3k.
[JEE 2011]
40. Let ω 6= 1 be a cube root of unity and S be the set of all non-singular matrices of the form
 
1 a b
 ω 1 c


ω 2 ω 1
2
where each of a, b, and c is either ω or ω . Then the number of distinct matrices in the set S is
(A) 2. (B) 6.
(C) 4. (D) 8.
[JEE 2011]
41. Let f : [−1, 2] → [0, ∞) be a continuous function such that f(x) = f(1 − x) for all x ∈ [−1, 2]. Let
2
Z
R 1 = f(x) dx, and R 2 be the area of the region bounded by y = f(x), x = −1, x = 2, and the
−1
x-axis. Then
(A) R 1 = 2R 2 . (B) R 1 = 3R 2 . (C) 2R 1 = R 2 . (D) 3R 1 = R 2 .

[JEE 2011]

2
42. Let f(x) = x and g(x) = sin x for all x ∈ R. Then the set of all x satisfying
(f ◦ g ◦ g ◦ f)(x) = (g ◦ g ◦ f)(x),

where (f ◦ g)(x) = f(g(x)), is
√ √
(A) ± nπ, n ∈ {0, 1, 2, . . .}. (B) ± nπ, n ∈ {1, 2, . . .}.
π
(C) + 2nπ, n ∈ {. . . , −2, −1, 0, 1, 2, . . .}. (D) 2nπ, n ∈ {. . . , −2, −1, 0, 1, 2, . . .}.
2

[JEE 2011]

43. Let f : (0, 1) → R be defined by
b − x
f(x) = ,
1 − bx

where b is a constant such that 0 < b < 1. Then
1
0
(A) f is not invertible on (0, 1). (B) f 6= f −1 on (0, 1) and f (b) = .
0
f (0)
1
0
(C) f = f −1 on (0, 1) and f (b) = . (D) f −1 is differentiable on (0, 1).
0
f (0)
[JEE 2011]