Page 14 - ISC-12

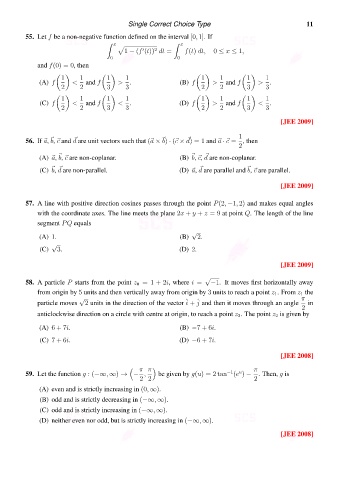
Single Correct Choice Type Jai Baba Ki11

55. Let f be a non-negative function defined on the interval [0, 1]. If
x x
Z Z
p
2
0
1 − (f (t)) dt = f(t) dt, 0 ≤ x ≤ 1,
0 0
and f(0) = 0, then

1 1 1 1 1 1 1 1
(A) f < and f > . (B) f > and f > .
2 2 3 3 2 2 3 3

1 1 1 1 1 1 1 1
(C) f < and f < . (D) f > and f < .
2 2 3 3 2 2 3 3
[JEE 2009]

1
~
~
~
~
56. If ~a, b, ~c and d are unit vectors such that (~a × b) · (~c × d) = 1 and ~a · ~c = , then
2
~
~
~
(A) ~a, b, ~c are non-coplanar. (B) b, ~c, d are non-coplanar.
~
~
~ ~
(C) b, d are non-parallel. (D) ~a, d are parallel and b, ~c are parallel.
[JEE 2009]
57. A line with positive direction cosines passes through the point P(2, −1, 2) and makes equal angles
with the coordinate axes. The line meets the plane 2x + y + z = 9 at point Q. The length of the line
segment PQ equals
√
(A) 1. (B) 2.
√
(C) 3. (D) 2.

[JEE 2009]
√
58. A particle P starts from the point z 0 = 1 + 2i, where i = −1. It moves first horizontally away

from origin by 5 units and then vertically away from origin by 3 units to reach a point z 1 . From z 1 the
√ π
ˆ
ˆ
particle moves 2 units in the direction of the vector i + j and then it moves through an angle in
2
anticlockwise direction on a circle with centre at origin, to reach a point z 2 . The point z 2 is given by
(A) 6 + 7i. (B) −7 + 6i.

(C) 7 + 6i. (D) −6 + 7i.

[JEE 2008]

π π π

u
−1
59. Let the function g : (−∞, ∞) → − , be given by g(u) = 2 tan (e ) − . Then, g is
2 2 2
(A) even and is strictly increasing in (0, ∞).
(B) odd and is strictly decreasing in (−∞, ∞).
(C) odd and is strictly increasing in (−∞, ∞).

(D) neither even nor odd, but is strictly increasing in (−∞, ∞).

[JEE 2008]